A new study suggests life may compute far faster — and deeper — than we ever imagined.



While some quantum computing companies push to demonstrate near-term commercial value, Rigetti Computing is taking a different approach. The company has identified specific technical milestones it said must be achieved before quantum systems can deliver meaningful business results, including 99.9% fidelity, 20-nanosecond gate speeds and real-time error correction.
In an interview we conducted at The Economist Commercializing Quantum event, Rigetti CEO Subodh Kulkarni outlined how the company’s novel chiplet-based architecture could help scale systems to 10,000 qubits, while also revealing an intriguing potential role for quantum computing in advancing artificial general intelligence.
Enter Quantum: What’s your take on the debate between return on investment versus technical capability in quantum computing?

The phenomenon of biological ultraweak photon emission (UPE), that is, extremely low-intensity emission (10 − 103 photons/cm2/sec) in the spectral range of 200 − 1,000 nm, has been observed in all living systems that have been examined. Here we report experiments that exemplify the ability of novel imaging systems to detect variations in UPE for a set of physiologically important scenarios. We use EMCCD and CCD cameras to capture single visible-wavelength photons with low noise and quantum efficiencies higher than 90%. Our investigation reveals significant contrast between the UPE from live vs. dead mice. In plants we observed that an increase in temperature and injuries both caused an increase in UPE intensity. Moreover, chemical treatments modified the UPE emission characteristics of plants, particularly the application of an anesthetic (benzocaine) to injury, which showed the highest emission among the compounds tested. As a result, UPE imaging provides the possibility of non-invasive label-free imaging of vitality in animals and the responses of plants to stress.
The authors have declared no competing interest.
Researchers at the University of Turku in Finland have developed a simple method to explore a complex area of quantum science. The discovery makes research in this field cheaper and more accessible, which could significantly impact the development of future laser, quantum and high-tech display technologies.
A team of researchers developed a new method for fabricating small structures known as optical microcavities. These structures allow scientists to study how light interacts with matter in a very precise process that can lead to the creation of novel quantum states called polaritons. Polaritons are unusual hybrid particles made from light and matter.
The results have been published in the journal Advanced Optical Materials.
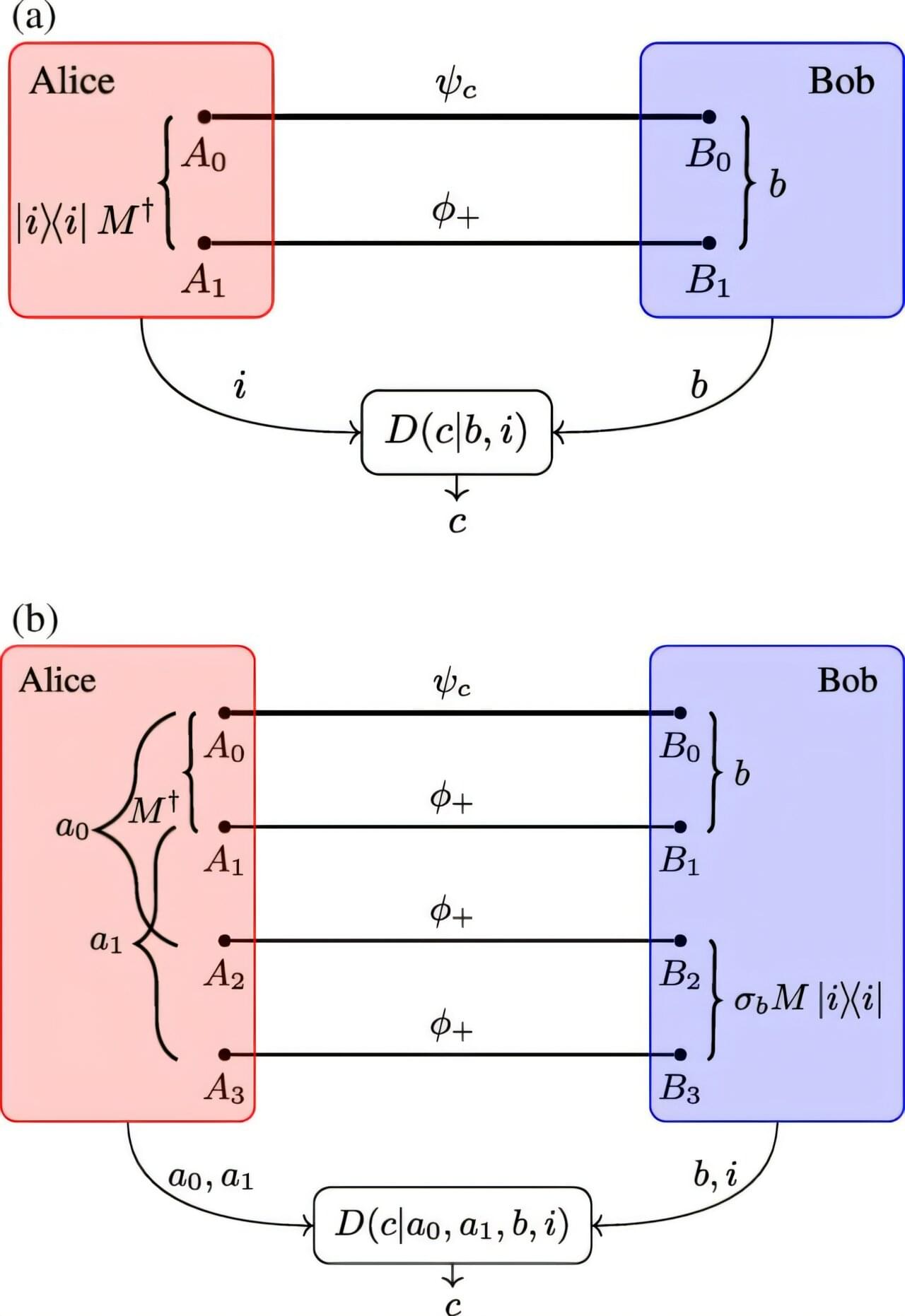
But such measurements are notoriously challenging: the instruments used are themselves governed by quantum laws, and their interaction with particles can alter the very properties they are meant to observe.
“The field of quantum measurements is still poorly understood because it has received little attention so far. Until now, research has mainly focused on the states of quantum systems themselves, which feature properties—like entanglement or superposition—that are more directly applicable to areas such as quantum cryptography or quantum computing,” explains Alejandro Pozas Kerstjens, Senior Research and Teaching Assistant in the Department of Applied Physics, Physics Section, at the UNIGE Faculty of Science.



A new study proposes that quantum information, encoded in entanglement entropy, directly shapes the fabric of spacetime, offering a fresh path toward unifying gravity and quantum mechanics.
Published in Annals of Physics, the paper presents a reformulation of Einstein’s field equations, arguing that gravity is not just a response to mass and energy, but also to the information structure of quantum fields. This shift, if validated, would mark a fundamental transformation in how physicists understand both gravity and quantum computing.
The study, published by Florian Neukart, of the Leiden Institute of Advanced Computer Science, Leiden University and Chief Product Officer of Terra Quantum, introduces the concept of an “informational stress-energy tensor” derived from quantum entanglement entropy.

A team of physicists has uncovered a surprising new way to explore one of science’s greatest challenges: uniting the two fundamental theories that explain how our universe works—Einstein’s theory of gravity and quantum mechanics.
Despite decades of effort, no one has fully explained how gravity—which governs massive objects like planets and stars—fits with quantum mechanics, which describes the behavior of the tiniest particles in the universe. But now, scientists believe light may hold the key.
Warner A. Miller, Ph.D., co-author and a professor in the Department of Physics at Florida Atlantic University’s Charles E. Schmidt College of Science in collaboration with scientists at the University of Seoul and Seoul National University, South Korea, found that light’s polarization —the direction it vibrates as it travels—can behave in an unexpected way when passing through curved space. Normally, this polarization shifts slightly due to the warping of space by gravity, a well-known effect.

An international team of researchers has successfully controlled the flow of energy in a molecule with the help of its pH value. The results of the study, led by Friedrich-Alexander-Universität Erlangen-Nürnberg (FAU), could contribute to the development of new sensors for medical diagnostics, for example.
The findings are also of interest for building more efficient solar cells and for use in quantum computing. The results have been published in the journal Nature Communications.
A process called singlet fission is at the center of the study. In future generations of solar cells, it should improve the utilization of light and thus increase efficiency. Until now, a large proportion of the energy that shines onto solar cells is lost and released as heat.