20th century physics has seen two major paradigm shifts in the way we understand Mother Nature. One is quantum mechanics, and the other is relativity. The marriage between the two, called quantum field theory, conceived an enfant terrible, namely anti-matter. As a result, the number of elementary particles doubled. We believe that 21st century physics is aimed at yet another level of marriage, this time between quantum mechanics and general relativity, Einstein’s theory of gravity. The couple has not been getting along very well, resulting in mathematical inconsistencies, meaningless infinities, and negative probabilities. The key to success may be in supersymmetry, which doubles the number of particles once more.
Why was anti-matter needed? One reason was to solve a crisis in the 19th century physics of classical electromagnetism. An electron is, to the best of our knowledge, a point particle. Namely, it has no size, yet an electric charge. A charged particle inevitably produces an electric potential around it, and it also feels the potential created by itself. This leads to an infinite “self-energy” of the electron. In other words, it takes substantial energy to “pack” all the charge of an electron into small size.
On the other hand, Einstein’s famous equation 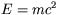 says that mass of a particle determines the energy of the particle at rest. For an electron, its rest energy is known to be 0.511 MeV. For this given amount of energy, it cannot afford to “pack” itself into a size smaller than the size of a nucleus. Classical theory of electromagnetism is not a consistent theory below this distance. However, it is known that the electron is at least ten thousand times smaller than that.
says that mass of a particle determines the energy of the particle at rest. For an electron, its rest energy is known to be 0.511 MeV. For this given amount of energy, it cannot afford to “pack” itself into a size smaller than the size of a nucleus. Classical theory of electromagnetism is not a consistent theory below this distance. However, it is known that the electron is at least ten thousand times smaller than that.






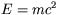 says that mass of a particle determines the energy of the particle at rest. For an electron, its rest energy is known to be 0.511 MeV. For this given amount of energy, it cannot afford to “pack” itself into a size smaller than the size of a nucleus. Classical theory of electromagnetism is not a consistent theory below this distance. However, it is known that the electron is at least ten thousand times smaller than that.
says that mass of a particle determines the energy of the particle at rest. For an electron, its rest energy is known to be 0.511 MeV. For this given amount of energy, it cannot afford to “pack” itself into a size smaller than the size of a nucleus. Classical theory of electromagnetism is not a consistent theory below this distance. However, it is known that the electron is at least ten thousand times smaller than that.